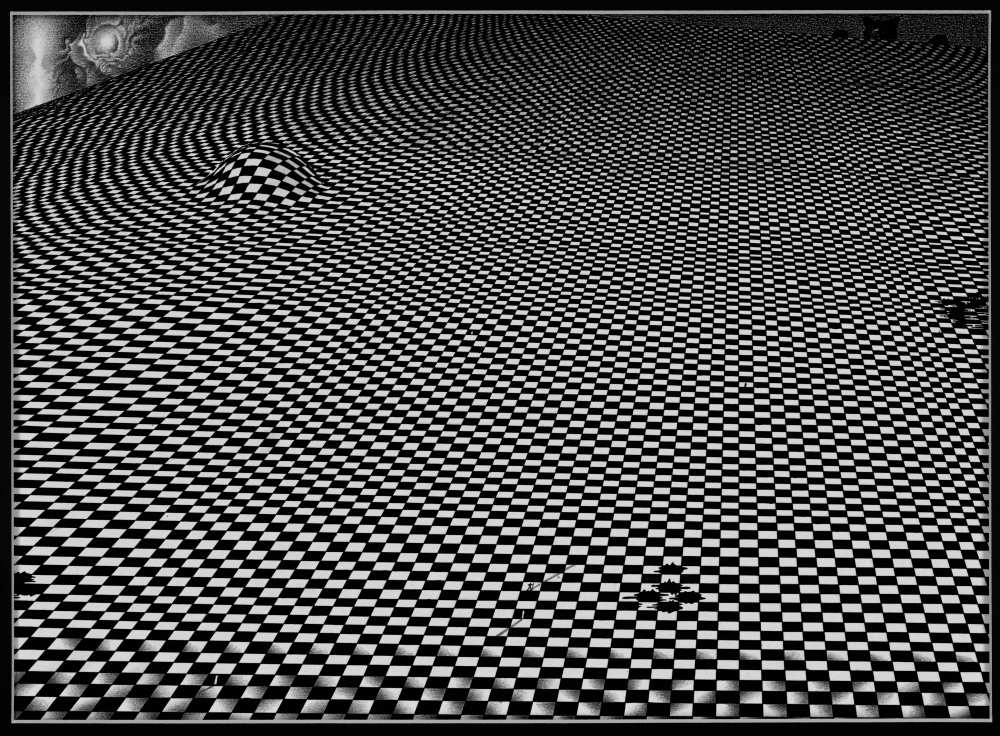
The Euclidean plane — the simplest minimal surface
N0. 119, 1974
(Minimal surfaces)
India ink and pencil on paper, 30.5x43 cm.
A vast checkered plane spreads out against a sky and into space, reaching outward in all directions, resisting a firm hold on the gravity that seeks to warp it out of shape. In a sense, it is a sea of squares. It is also a surface locally perturbed by a swell, an undulation that has increased its area, a fact one can see in the larger squares of the perturbation zone. All of the squares appeared the same before being perturbed, and yet the perturbation causes some to grow. Remarkable too is the plane's ability to keep the same area despite any perturbations, no matter how large the amplitude. Yet, some minimal surfaces, more complicated than a plane, will decrease their area if the amplitude of the perturbation is small, but has large support—for instance, a two-dimensional equator on a three-dimensional sphere.