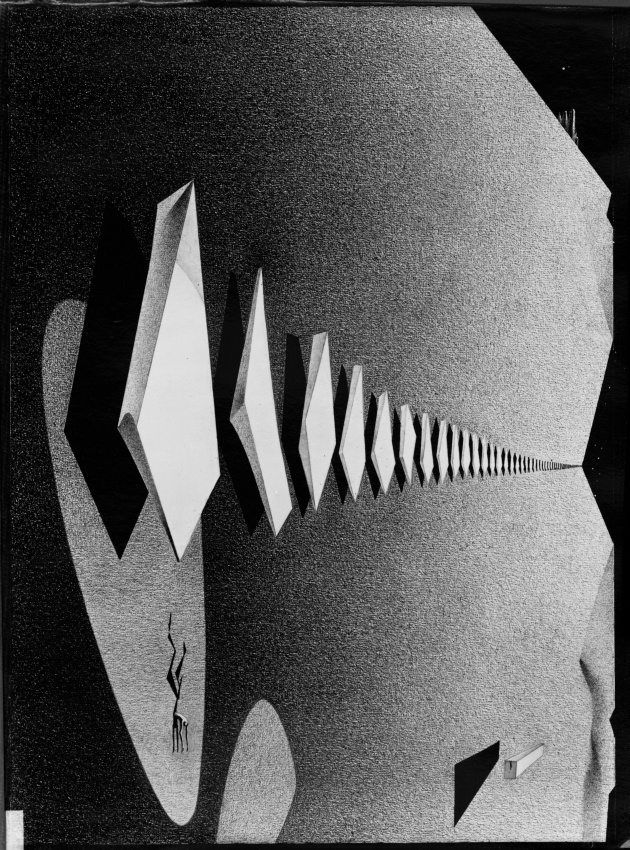
An orbit of the action of an infinite group
No. 170, 1975
(Group actions)
India ink and pencil on paper, 31.5x44 cm.
Suspended slate steps span a great desert distance, flowing smoothly across a vast sandy plane, between flattened dunes, under the heat of a ferocious midday star. See here an object, one associated with the action of a group, an action given on a space where the elements of the group are homeomorphisms of the space. Consider too an orbit of some infinite subgroup of the given group. If we picture some domain in the space as a single diamond shape—a three-dimensional polyhedron—and apply to it successively all the elements of the infinite subgroup, the result is an infinite sequence of polyhedra duplicating one another. This we can call the orbit of the polyhedron under the action of the subgroup. If images of the polyhedron regularly cover the whole space, only intersecting at their boundaries, then we have a fundamental region of the given subgroup. Note too that not all of the polyhedra are identical. Each differs slightly from its neighbor. This is okay. Why? Because they are roughly similar to one another by way of a homeomorphism.