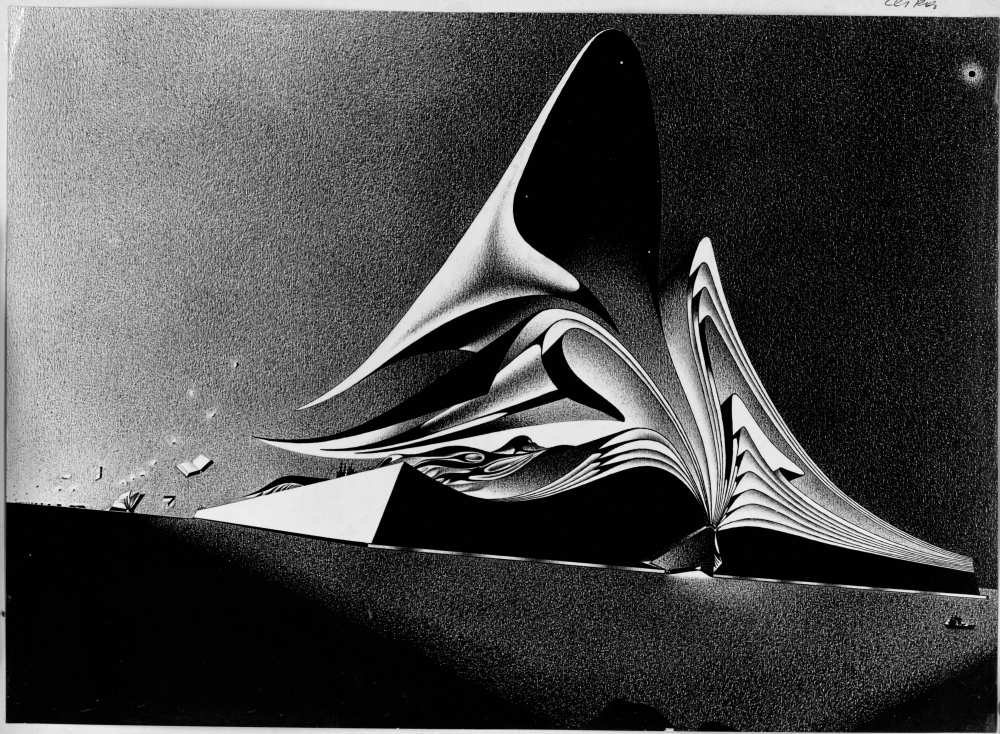
Singularities of smooth functions
No. 180, 1976
(Mathematical analysis and geometry, theory of singularities)
India ink and pencil on paper, 31.5x44 cm.
Spread over a truly surreal literary landscape, giant books blow in the wind, their pages twisting and turning in ways that paper and gravity do not ordinarily allow. A star shines crisply from above, although a hurricane is on the way. Within this image are several singularities which can arise from a smooth mapping. Singularities are very intriguing entities, arising in so many areas of mathematics and physics, ranging from celestial mechanics to optics to wave theory. In the theory of singularities of differentiable mappings, various types of singularities can be classified within the natural equivalences generated by regular changes of coordinates. For instance, in the right-hand pages of the books shown, we see objects that reflect the work of mappings. A point, a fold, and a gather, for instance, are the only stable singularities—ones not destroyed by small perturbations.