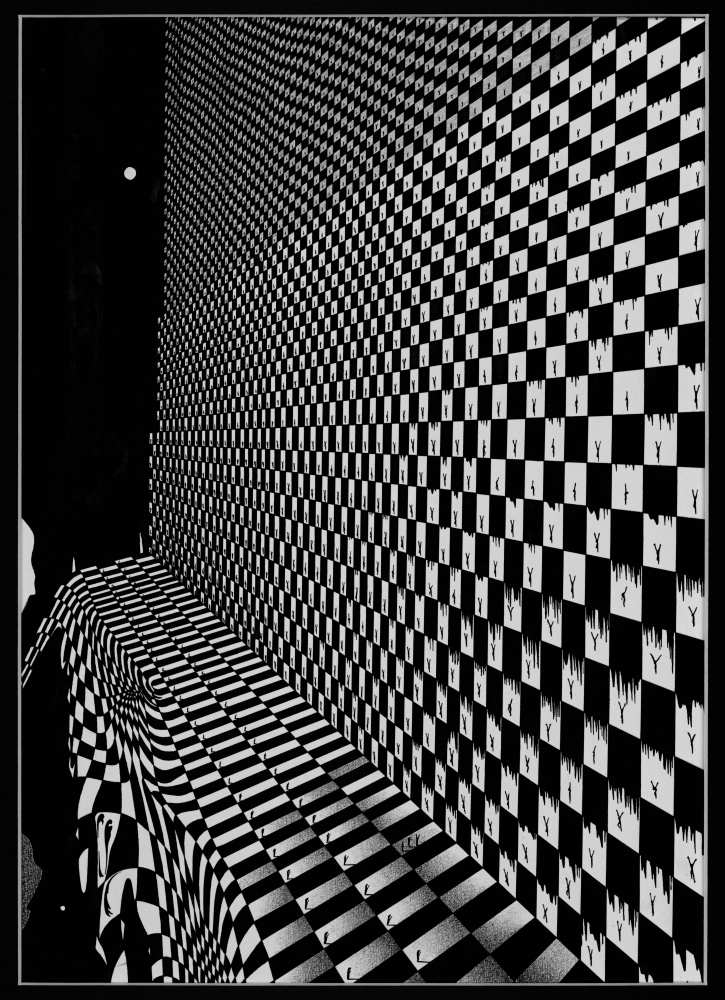
Simplicial, cubic, cellular chains
No. 136, 1974
(Homology theory)
India ink on paper, 20.5x43 cm.
In this world of walls, squares hang on warped planes that roll off into space and exist as small universes for the figures that occupy them. In fact, each square is part of a larger polyhedron, which has been partitioned so that each square is assigned a number, represented here in the form of individual human beings. Each human has a unique posture, which corresponds to a certain coefidcient. From this collection of figures, one can compute a boundary chain, which requires summing up all of the segments that comprise the boundaries of the squares, adding up the coefficients. If the sum is zero, then the chain is called a cycle. In the case of this chain, it is not a cycle. In general, this image points to the topological idea of simplicial, or cellular, chains, which underlie a procedure for computing topological invariants of spaces, namely, the homology and cohomology groups.