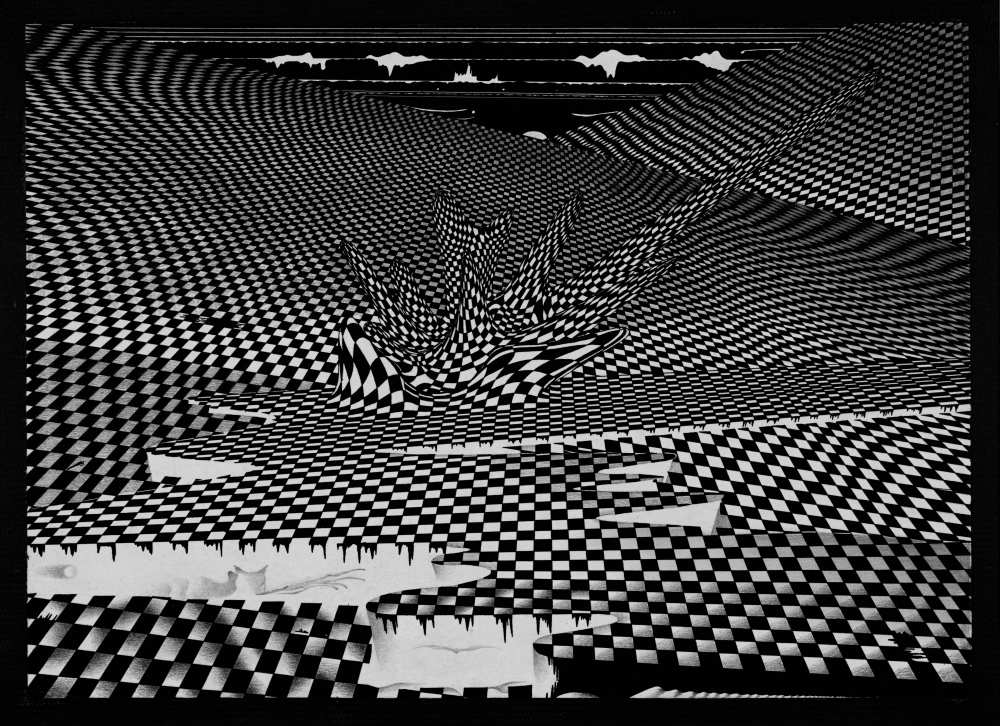
Algebraic surfaces of higher order and the simplicial approximation theorem
No. 120, 1974
(Topology and function theory)
India ink and pencil on paper, 30x43 cm.
Out ofthis broad and rolling surface, a virtual checkerboard landscape of sand dunes, cracked into pieces in places, grows a garden of mathematical forms. The picture also shows a surface finely partitioned into squares, and each of those squares can be thought of as partitioned into triangles, ad infinitum. Since the surface is so much larger than the squares, assume that a linear mapping function applies. Observe too that the graphs thin peaks correspond to points where the original smooth mapping has greatest curvature. In a neighborhood of such points, one must partition the surface into finer squares to get a piecewise linear approximation. The greater the curvature of the surface, the smaller the size of the square, something that is especially visible around the peaks. Indeed, we can always approximate an arbitrary continuous mapping of polyhedra with a piecewise linear mapping homotopic to the original.